Details About The Arithmetic Mean And Arithmetic Progression
If expressed in terms of statistics, then the arithmetic mean is the proportion of the summation of all the observations to the total number of observations. The average income of the family, the average amount of rainfall, and many more can be considered as an example of an arithmetic mean. In more simple terms, arithmetic mean can be considered as an average of something.
What does the understanding behind arithmetic mean?
All the numbers in the data set are summed up and then divided by the total number of observations that are present within the set. It has been seen that the arithmetic means of the distribution equalize the middlemost value whenever the total number of the distribution is even. Different methods are applied while calculating the arithmetic mean.
Let us understand the concept behind arithmetic means. Suppose there is a number distribution that consists of numbers, 7,9,6,4. Then the summation of the items present in the number distribution is (7+7+6+4=24) and the total number of items present in the distribution is 4. The arithmetic mean of the distribution is the summation of the items divided by the total number of items, i.e., (24/4=6). Hence, the arithmetic mean of the above distribution is 6.
The formula for the arithmetic mean
Mean=Sum of all item/Number of item
Properties of arithmetic mean
- If the observation of the data set has a similar value for example say ‘n’ the arithmetic mean of the data set will be ‘n’. Let’s understand this with an example. Say a data set consists of 16 as all four values of the observation. Then the arithmetic mean is 16, because, 16+16+16+16 =64, and 64/4 =16.
- The sum of the difference between the observation and the arithmetic mean is always equal to zero. (a1-ā)+(a2-ā)+…+(an+ā)=0
- One of the interesting properties of arithmetic means is if any of the observations of the data set gets increased or gets lowered by a particular value then the arithmetic mean also gets boosted up or gets lowered by that particular value.
- If any observation of the data set gets multiplied or divided by a particular value then according to the property the mean of the data set will also get multiplied or divided by the particular value.
What is the understanding behind arithmetic progression?
Arithmetic progression is a consistent series of numbers where the difference between every two consecutive numbers is similar. In other words, every number in the arithmetic progression can be obtained by adding a fixed value to its preceding number. Consider the following example to gain a detailed understanding of the arithmetic progression.
- 2,4,6,8…
Here,
a(first number)= 2,
d(common difference)= 2.
In this example, it can be seen that every number in the entire series can be obtained by adding 2 to its proceedings number.
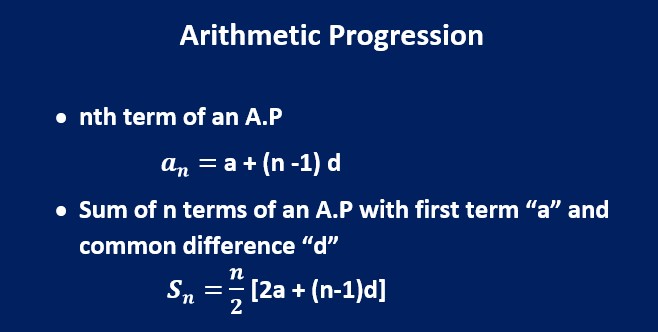
The formula for calculating the value of the summation of the numbers present in the series.
There are two ways of calculating the sum.
- This formula can be used to calculate the sum of the values whenever the nth term of the series is not known.
Sn=n/2[2a+(n-1)d]
- This formula can be used when any term of the series is known.
Sn= n/2[a1+an]
Other important formulae
- Common difference = a2-a1
- nth term = a +( n-1)d
Conclusion
Arithmetic mean and arithmetic progression are the topics on which one needs to gain intensive knowledge to flourish in the field of mathematics. But to gain knowledge, it is essential to take the advice of an expert who can show you the right path. You can take the suggestion from cuemath. Cuemath has an expert who can provide lessons on any chapter of mathematics with efficiency and accuracy. If you have any questions about the concept of any mathematics chapter, you can seek help from cuemath because the professionals will use advanced methods to resolve your concerns.